考虑惯性力矩与剪切变形的曲梁面内自由振动微分方程的建立

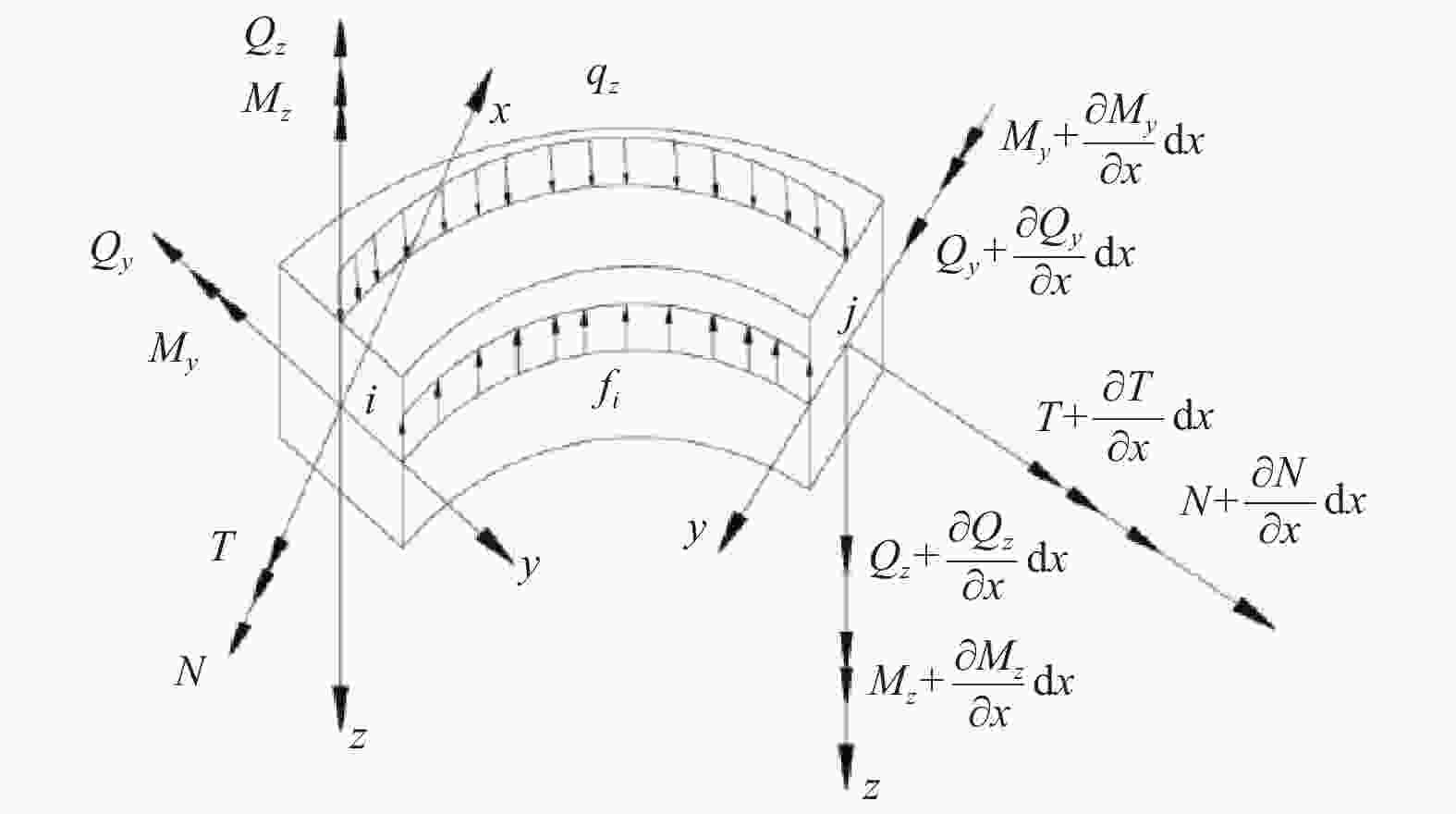
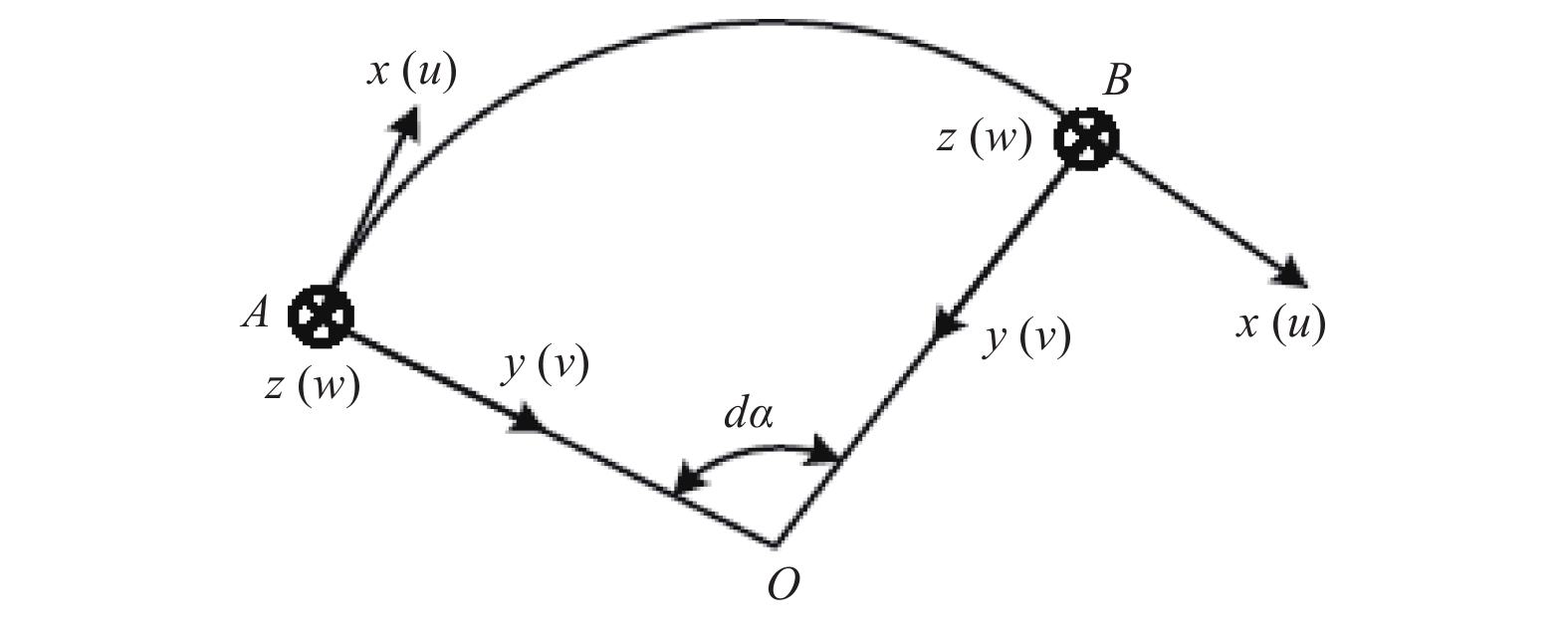
Establishment of differential equation of in-plane free vibration of curved beams under MOI and shear deformation

School of Urban Rail Transportation, Shanghai University of Engineering Science, Shanghai 201620, China

摘要: 振动微分方程的推导与建立是结构动力分析的关键. 依据Timoshenko梁理论，计入惯性力矩与剪切变形的影响，通过联立几何变形协调方程与内力平衡方程，推导建立曲梁面内横向弯曲自由振动微分方程与曲梁面内轴向自由振动微分方程. 研究结果为曲梁动力学研究提供一定的理论基础.
- 曲梁 /
- 惯性力矩 /
- 剪切变形 /
- 振动微分方程 /
- Timoshenko梁
Abstract: The derivation and establishment of vibration differential equation are the key to the structural dynamic analysis. The moment of inertia (MOI) and shear deformation were taken into account, the differential equations of in-plane free vibration about lateral bending and axial deformation of curved beams were derived and established by combining geometric equations and the internal force equilibrium according to the theory of Timoshenko beam. The research results can provide a theoretical basis for dynamic study on the curved beams.
- curved beams /
- moment of inertia (MOI) /
- shear deformation /
- vibration differential equation /
- Timoshenko beam

图 1 曲梁平面图

Figure 1. Plan of the curved beam

下载: 全尺寸图片幻灯片

图 2 曲梁微段内力图

Figure 2. Graph of curved beam segment internal force

下载: 全尺寸图片幻灯片

[1]	叶康生, 殷振炜. 平面曲梁面内自由振动有限元分析的p型超收敛算法[J] . 工程力学,2019,36(5):28 − 36,52.
[2]	叶康生, 梁童. 平面曲梁面外自由振动有限元分析的p型超收敛算法[J] . 工程力学,2020,37(10):17 − 27. doi: 10.6052/j.issn.1000-4750.2019.11.0694
[3]	陈明飞, 靳国永, 张艳涛, 等. 弹性约束的功能梯度曲梁等几何振动分析[J] . 振动工程学报,2020,33(5):930 − 939.
[4]	李星照, 李朋洲, 孙磊. 曲梁自由振动微分方程的解耦解法及验证[J] . 核动力工程,2016,37(S2):7 − 10.
[5]	宋郁民, 吴定俊, 李奇. 圆弧曲梁振动微分方程推导及振动特性分析[J] . 沈阳建筑大学学报(自然科学版),2012,28(3):400 − 404.
[6]	宋郁民. 曲梁振动微分方程组求解[C]//第23届全国结构工程学术会议论文集(第Ⅰ册). 兰州: 中国力学学会, 中国力学学会《工程力学》编委会, 兰州理工大学, 2014: 258-264.
[7]	包世华. 结构动力学[M]. 武汉: 武汉理工大学, 2017: 105-107.
[8]	李卓庭, 宋郁民. 曲梁几何方程推导[J] . 工程力学,2019,36(S1):12 − 16.