含边界层的机械臂分数阶滑模控制

刘世杰; 黄志来; 杨明星; 徐培民

含边界层的机械臂分数阶滑模控制

刘世杰^{a, b,},
黄志来^{a, b, ,},
杨明星^{a, b},
徐培民^{a, b}

a.
安徽工业大学特种重载机器人安徽省重点实验室, 马鞍山 243000
b.
机械工程学院, 马鞍山 243000

基金项目: 国家自然科学基金资助项目(52005006)

详细信息

作者简介:
刘世杰(1996−)，男，在读硕士，研究方向为动力学及控制. E-mail: liushijie9687@163.com

通讯作者:
黄志来(1983−)，男，讲师，博士，研究方向为动力学及控制. E-mail: zhilai_huang@foxmail.com

中图分类号: TP241
计量
- 文章访问数: 609
- HTML全文浏览量: 518
- PDF下载量: 361
- 被引次数: 0
出版历程
- 收稿日期: 2021-08-31
- 刊出日期: 2022-02-23

Fractional-order sliding mode control of robotic arms with boundary layer

LIU Shijie^{a, b
,},
HUANG Zhilai^{a, b
, ,},
YANG Mingxing^{a, b},
XU Peimin^{a, b}

a.
Anhui Province Key Laboratory of Special Heavy Load Robot
b.
School of Mechanical Engineering, Anhui University of Technology, Ma’anshan 243000, China

摘要

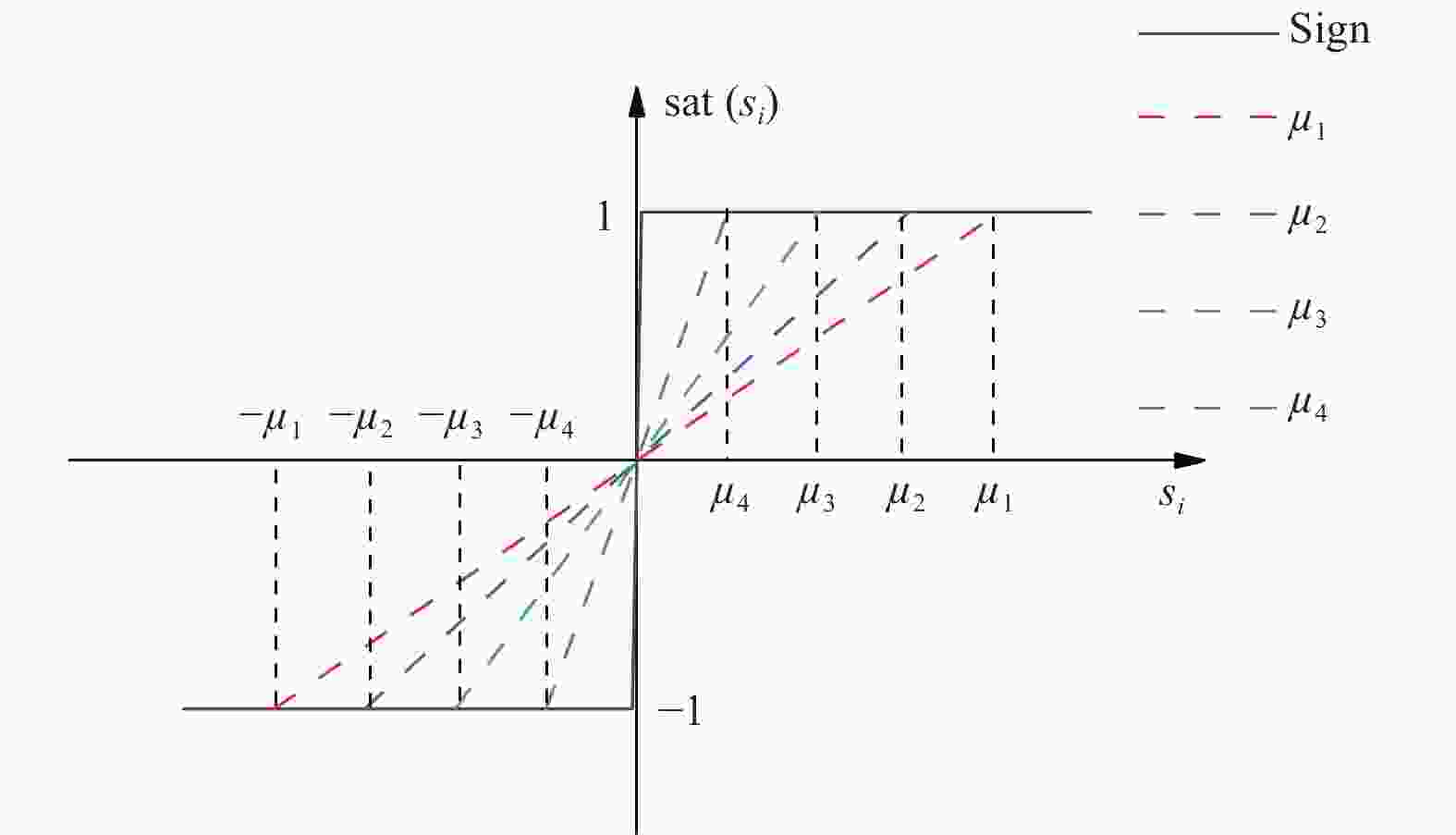
摘要: 针对含扰动的机械臂系统，在经典滑模控制基础上引入分数阶微积分构造分数阶滑模面，同时在趋近律中设置边界层，用饱和函数替代符号函数以削弱滑模面的抖振现象，从而获得一种含边界层的机械臂分数阶滑模控制，并通过李雅普诺夫直接法证明其收敛性. 算例以两自由度机械臂系统为被控对象，仿真结果表明，相比于经典的滑模控制，含边界层的机械臂分数阶滑模控制可通过调节微分阶次获得更好的收敛性，实现精确的轨迹跟踪.
- 滑模控制 /
- 边界层法 /
- 饱和函数 /
- 分数阶微积分 /
- 机械臂
Abstract: For the robotic arm system with perturbation, the fractional-order calculus was introduced to construct a fractional-order sliding mode surface based on classical sliding mode control. While a boundary layer had set in the reaching law, and the sign function was replaced with the saturation function to weaken chattering phenomenon of the sliding mode surface to obtain a fractional-order sliding mode control of robotic arms with boundary layer, and its convergence had proved by the direct method of Lyapunov. Example with a two-degree-of-freedom robotic arm system as the controlled object, the simulation result shows that the fractional-order sliding mode control of robotic arms with boundary layer can achieve better convergence and accurate trajectory tracking by adjusting the differential order compared with the classical sliding mode control.
- sliding mode control /
- boundary layer method /
- saturation function /
- fractional-order calculus /
- robotic arm

HTML全文

图 1 饱和函数与符号函数

Figure 1. Saturation function and sign function

下载: 全尺寸图片幻灯片

图 2 轨迹跟踪

Figure 2. Trajectory tracking

下载: 全尺寸图片幻灯片

图 3 轨迹跟踪误差

Figure 3. Trajectory tracking error

下载: 全尺寸图片幻灯片

表 1 系统到达稳态时间

Table 1. System reaching steady state time

关节 SMC /s FOSMC /s
α=0.2 α=0.4 α=0.6 α=0.8

1 1.44 0.79 1.20 1.30 1.73
2 1.36 0.86 0.88 1.03 1.47

下载: 导出CSV

参考文献(29)

[1]	BAGLEY R L. On the fractional calculus model of viscoelastic behavior[J] . Journal of Rheology,1998,30(1):133 − 155.
[2]	KOBER H. On fractional integrals and derivatives[J] . Quarterly Journal of Mathematics,1940(1):193 − 211.
[3]	IGOR P. Fractional-order systems and PIλDμ controllers[J] . IEEE Trans. Automatic Control,1996,44(1):208 − 214. doi: 10.1109/9.739144
[4]	WANG L N, CHANG H, LI Y. Dynamics analysis and chaotic control of a fractional-order three-species food-chain system[J] . Mathematics,2020,8(3):409 − 15. doi: 10.3390/math8030409
[5]	ERAY O, TOKAT S. The design of a fractional-order sliding mode controller with a time-varying sliding surface[J] . Transactions of the Institute of Measurement and Control,2020,42(16):3196 − 3215. doi: 10.1177/0142331220944626
[6]	LI Z Q, CHEN L Q, ZHENG Q, et al. Control of a path following caterpillar robot based on a sliding mode variable structure algorithm[J] . Biosystems Engineering,2019,186:293 − 306. doi: 10.1016/j.biosystemseng.2019.07.004
[7]	PILLONI A, PISANO A, USAI E. Robust finite-time frequency and voltage restoration of inverter-based microgrids via sliding-mode cooperative control[J] . IEEE Transactions on Industrial Electronics,2018,65(1):907 − 917. doi: 10.1109/TIE.2017.2726970
[8]	BOUKADIDA W, BENAMOR A, MESSAOUD H, et al. Metaheuristics-based multi-objective design of global robust optimal sliding mode control of discrete uncertain systems[J] . International Journal of Control, Automation and Systems,2019,17(6):1378 − 1392. doi: 10.1007/s12555-018-0486-y
[9]	ZHANG X, ZHANG J, LU W R, et al. Research on fractional sliding mode synchronous control of robotic ARMS under uncertain disturbance[J] . Automatic Control and Computer Sciences,2021,55(1):26 − 37. doi: 10.3103/S0146411621010107
[10]	DUC T M, HOA N V, DAO T P. Adaptive fuzzy fractional-order nonsingular terminal sliding mode control for a class of second-order nonlinear systems[J] . Journal of Computational and Nonlinear Dynamics,2018,13(3):031004.
[11]	WANG J, SHAO C, CHEN Y Q. Fractional order sliding mode control via disturbance observer for a class of fractional order systems with mismatched disturbance[J] . Mechatronics,2018,53:8 − 19. doi: 10.1016/j.mechatronics.2018.05.006
[12]	HUANG S H, XIONG L Y, WANG J, et al. Fixed-time fractional-order sliding mode controller for multimachine power systems[J] . IEEE Transactions on Power Systems,2021,36(4):2866 − 2876.
[13]	NGUYEN S D, LAM B D, NGO V H. Fractional-order sliding-mode controller for semi-active vehicle MRD suspensions[J] . Nonlinear Dynamics,2020,101(3):1 − 10.
[14]	MA H F, LIU C, LIU Y, et al. Sliding mode control for uncertain discrete-time systems based on fractional order reaching law[J] . IET Control Theory & Applications,2019,13(13):1963 − 1970.
[15]	BABAEI S M, YAHYAZADEH M, MARJ H F. Novel MPPT for linear-rotational sun-tracking system using fractional fuzzy grey-based sliding mode control[J] . Iranian Journal of Science and Technology, Transactions of Electrical Engineering,2020,44(4):1379 − 1401. doi: 10.1007/s40998-020-00324-6
[16]	RIGATOS G G. Control and disturbances compensation in underactuated robotic systems using the derivative-free nonlinear Kalman filter[J] . Robotica,2017,35(3):687 − 711. doi: 10.1017/S0263574715000776
[17]	TAVARES A, MADRUGA S P, BRITO A V, et al. Dynamic leader allocation in multi-robot systems based on nonlinear model predictive control[J] . Journal of Intelligent and Robotic Systems,2019,98(6):1 − 18.
[18]	SU H, HU Y B, KARIMI H R, et al. Improved recurrent neural network-based manipulator control with remote center of motion constraints: Experimental results[J] . Neural Networks,2020,131:291 − 299. doi: 10.1016/j.neunet.2020.07.033
[19]	DAI L, YU Y, ZHAI D H, et al. Robust model predictive tracking control for robot manipulators with disturbances[J] . IEEE Transactions on Industrial Electronics,2021,68(5):4288 − 4297. doi: 10.1109/TIE.2020.2984986
[20]	KOMIJANI H, MASOUMNEZHAD M, ZANJIREH M M, et al. Robust hybrid fractional order proportional derivative sliding mode controller for robot manipulator based on extended grey wolf optimizer[J] . Robotica,2020,38(4):605 − 616. doi: 10.1017/S0263574719000882
[21]	YIN C, CHENG Y H, ZHONG S M, et al. Fractional-order switching type control law design for adaptive sliding mode technique of 3D fractional-order nonlinear systems[J] . Complexity,2016,21(6):363 − 373. doi: 10.1002/cplx.21696
[22]	RAHIMKHANI P, ORDOKHANI Y, BABOLIAN E. A new operational matrix based on Bernoulli wavelets for solving fractional delay differential equations[J] . Numerical Algorithms,2017,74(1):223 − 245. doi: 10.1007/s11075-016-0146-3
[23]	MADDAHI A, SEPEHRI N, KINSNER W. Fractional-Order Control of Hydraulically Powered Actuators: Controller Design and Experimental Validation[J] . IEEE/ASME Transactions on Mechatronics,2019,24(2):796 − 807. doi: 10.1109/TMECH.2019.2894105
[24]	WANG Y Q, FENG Y T, ZHANG X G, et al. A new reaching law for antidisturbance sliding-mode control of PMSM speed regulation system[J] . IEEE Transactions on Power Electronics,2020,35(4):4117 − 4126. doi: 10.1109/TPEL.2019.2933613
[25]	BRAHMI B, LARAKI M H, BRAHMI A, et al. Improvement of sliding mode controller by using a new adaptive reaching law: Theory and experiment[J] . ISA Transactions,2020,97:261 − 268. doi: 10.1016/j.isatra.2019.08.010
[26]	HOU S X, CHU Y D, FEI J T. Robust intelligent control for a class of power-electronic converters using neuro-fuzzy learning mechanism[J] . IEEE Transactions on Power Electronics,2021,36(8):9441 − 9452. doi: 10.1109/TPEL.2021.3049553
[27]	TAHERI, FERDOWSI E, DANESH M H, et al. Design boundary layer thickness and switching gain in SMC algorithm for AUV motion control[J] . Robotica,2019,37(10):1785 − 1803. doi: 10.1017/S0263574719000262
[28]	CRISTOFAR A. Robust distributed control of quasilinear reaction-diffusion equations via infinite-dimensional sliding modes[J] . Automatica,2019,104:165 − 172. doi: 10.1016/j.automatica.2019.02.039
[29]	GUO Y, WOO P Y. An adaptive fuzzy sliding mode controller for robotic manipulators[J] . Systems Man & Cybernetics Part A Systems & Humans IEEE Transactions on,2003,33(2):149 − 159.