Multi-objective optimization of PMSM rotor structure based on stratified optimization strategy
-
摘要:
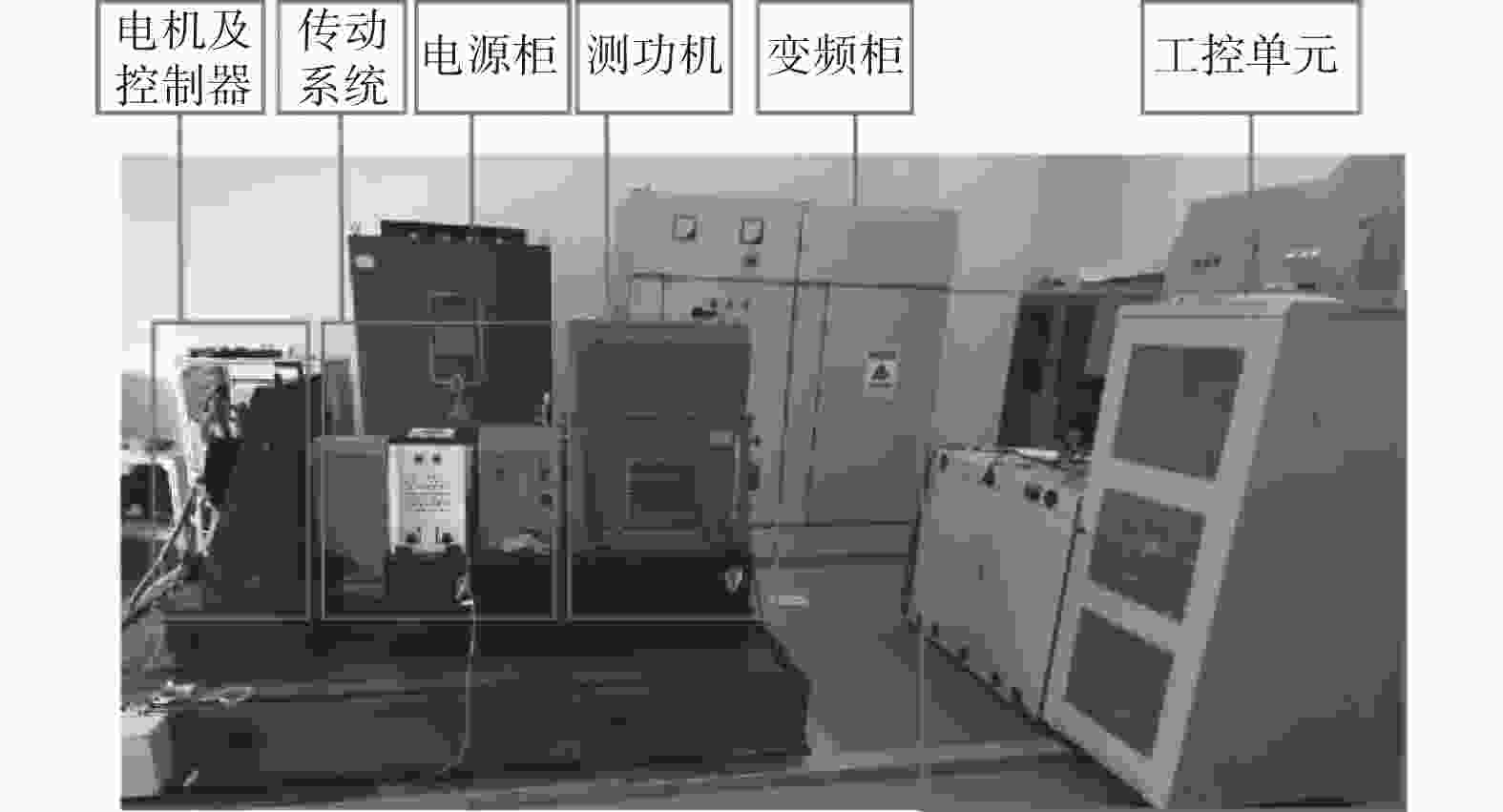
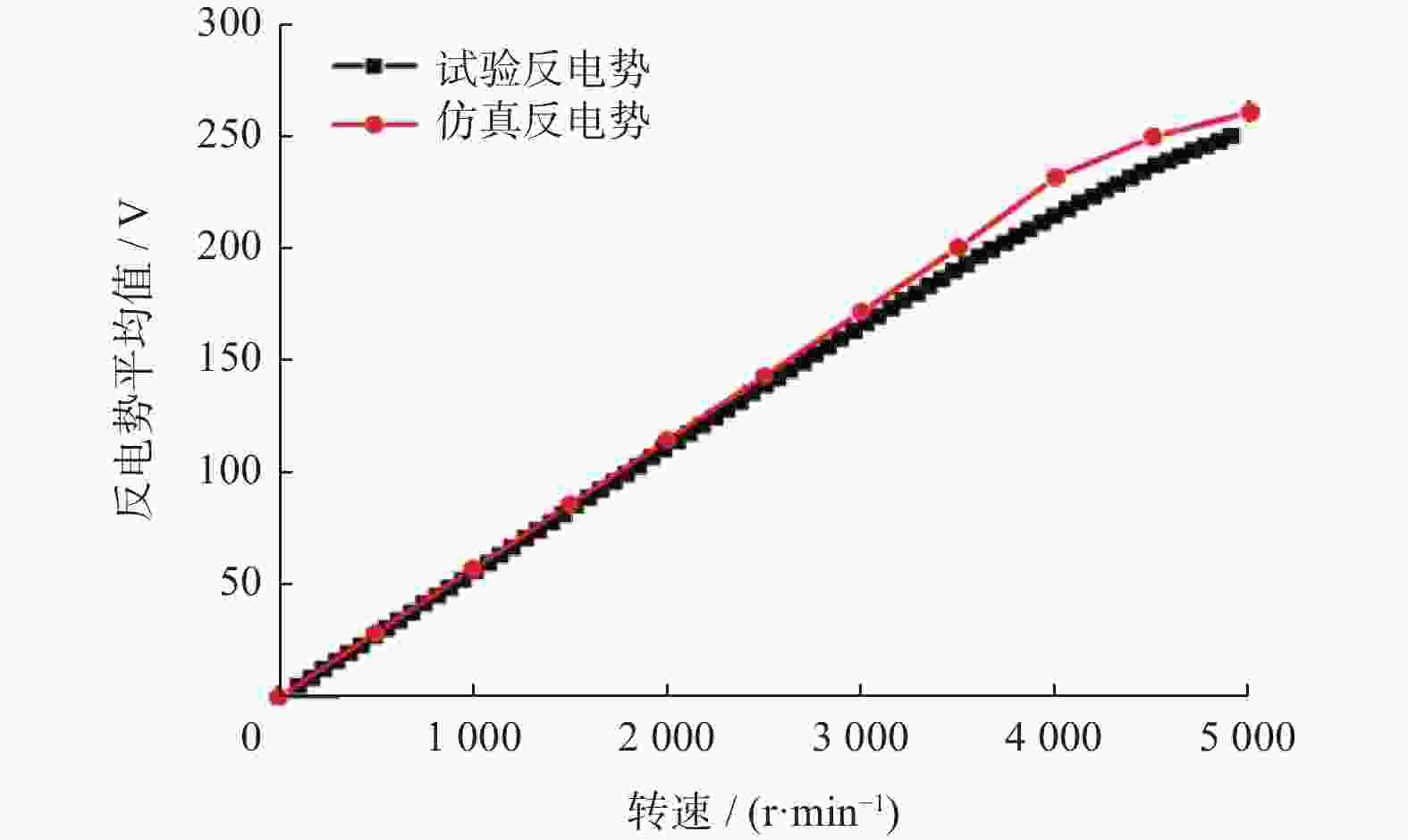
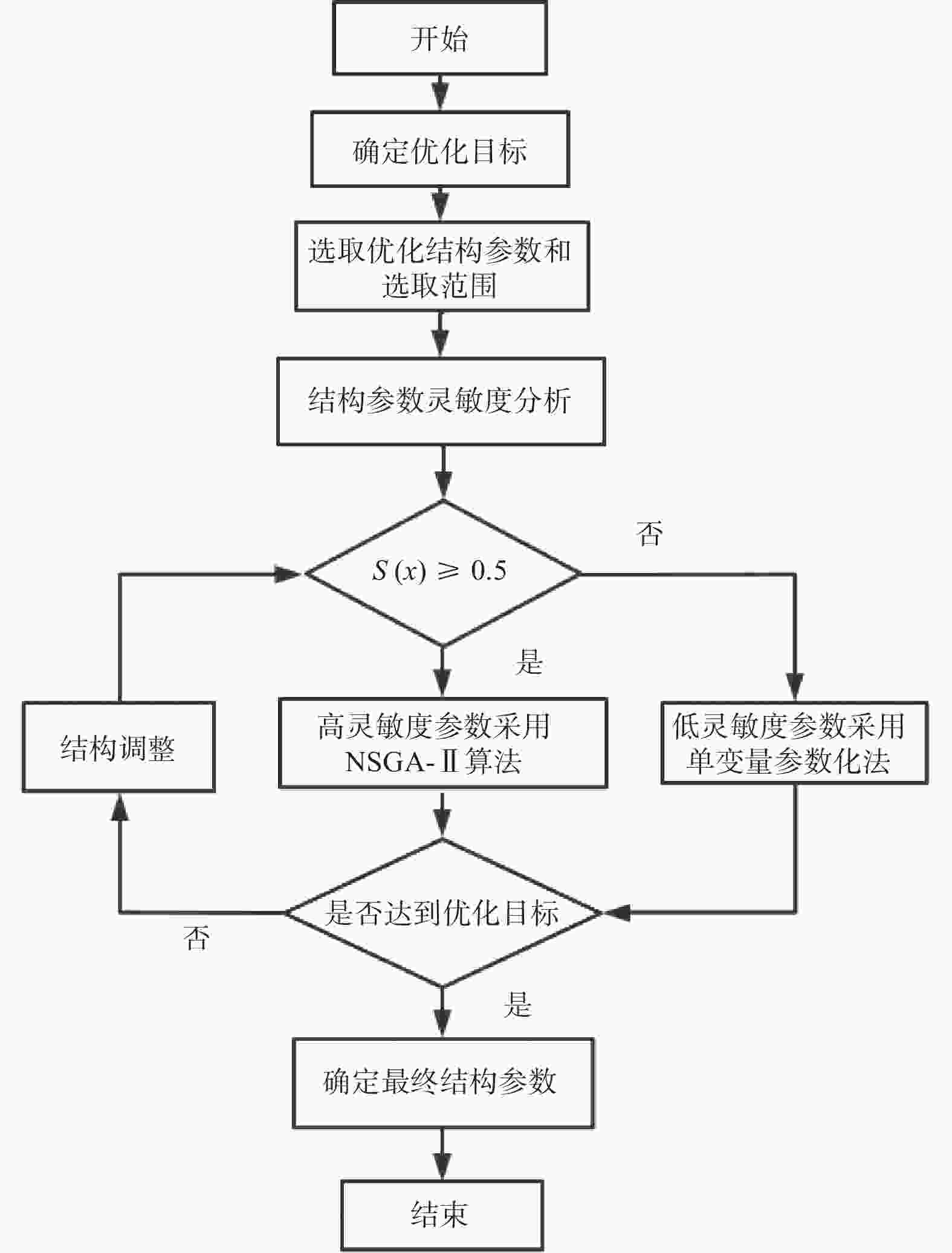
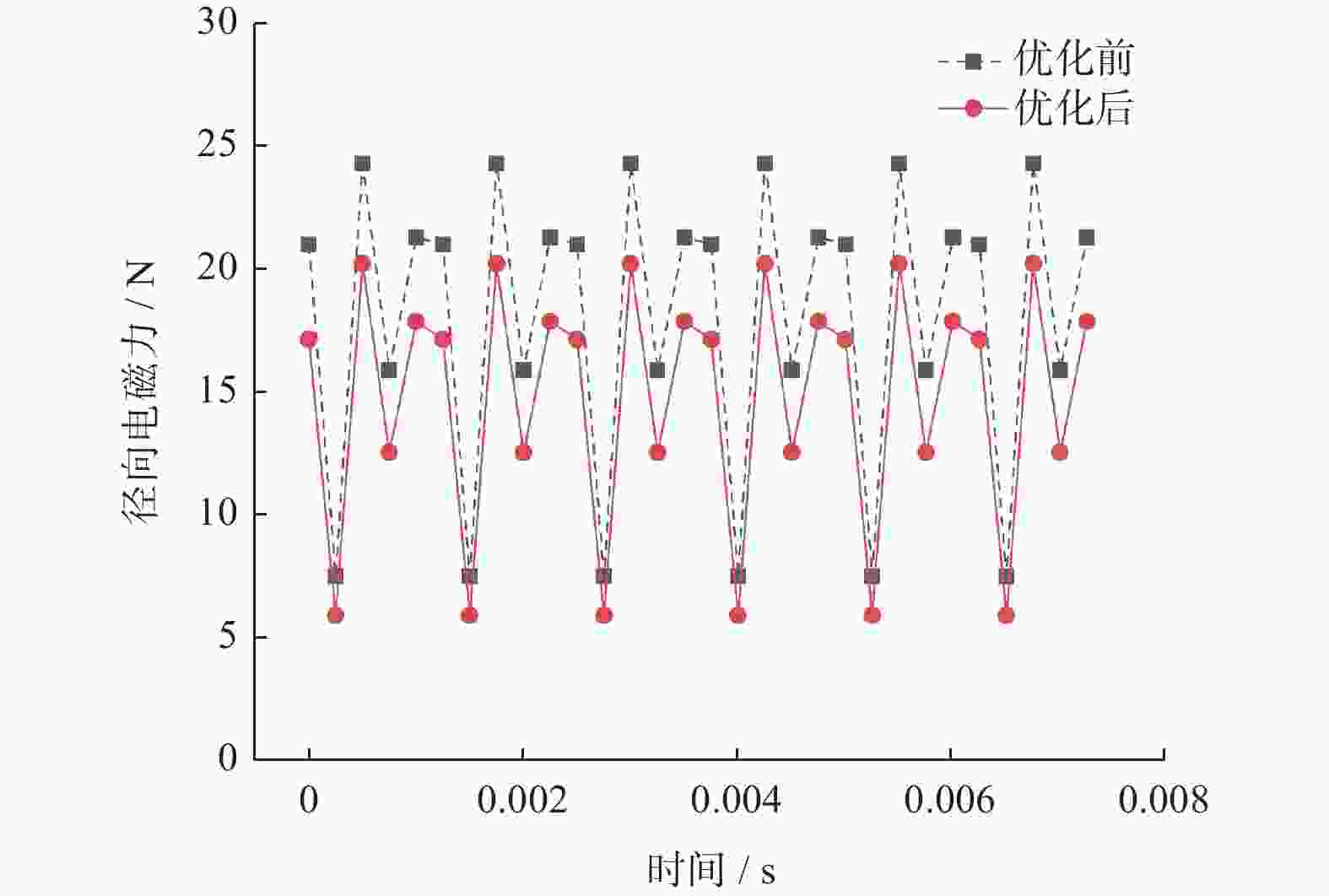
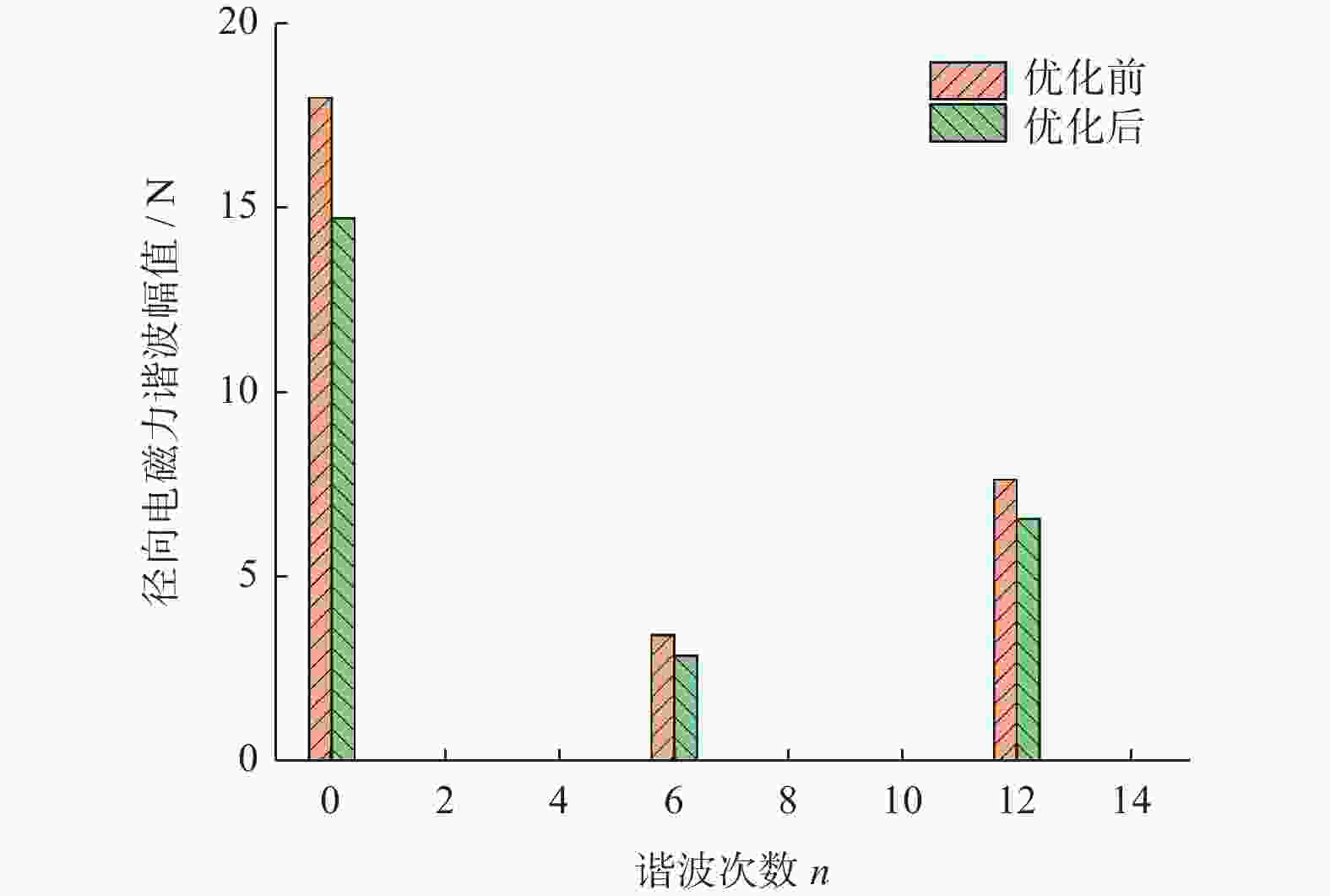
永磁同步电机对转矩输出的平滑性有较高要求. 内置式永磁同步电机受齿槽转矩、磁阻转矩脉动、电磁转矩脉动等因素影响，输出转矩脉动相对较大，增加了电磁噪声和输出的不稳定性. 基于一台20 kW永磁同步电机建立有限元模型，验证模型的准确性. 以电机最大输出转矩、最小转矩脉动、最小齿槽转矩为优化目标，对电机转子结构参数进行灵敏度分析，并综合NSGA−Ⅱ算法和单变量参数法进行分层优化. 与单层优化相比，分层优化对易受变量影响的目标提升效果更好. 声学仿真验证了优化后的转子结构对电磁噪声改善效果显著.
Abstract:The permanent magnet synchronous motor (PMSM) has a high requirement on the smoothness of torque output. Due to influences of groove torque, reluctance torque pulsation, electromagnetic torque pulsation and other factors, the output torque pulsation of the interior PMSM is relatively large, which increases electromagnetic noise and output instability. A finite element model was established based on a 20 kW PMSM, and the accuracy of the model was verified. Taking the maximum output torque, the minimum torque pulsation and the minimum slot torque as optimization objectives, the sensitivity analysis of rotor structure parameters was carried out, and the stratified optimization was carried out by combining NSGA−Ⅱ algorithm and single variable parameter method. Compared with single-layer optimization, stratified optimization has a better effect on the target which is susceptible to variables. The acoustic simulation shows that the optimized rotor structure can improve electromagnetic noise significantly.
-
图 3 试验与仿真空载反电势对比
Figure 3. Comparison between test and simulated no-load counter electromotive force
图 6 高灵敏度参数优化3D散点图
Figure 6. Optimized 3D scatter plot with high sensitivity parameters
图 12 电机径向电磁力时间谐波分解
Figure 12. Time harmonic decomposition of radial electromagnetic force of motor
表 1 样机参数
Table 1. Motor parameters
电机参数 参数值 极数 8 定子槽数 48 定子外径/mm 215 永磁体厚度/mm 4 额定电压/V 252 额定转速/(r•min−1) 2 000 气隙长度/mm 0.8 绕组类型 星型连接 
下载: 导出CSV
表 2 电机主要结构参数初值及变化范围
Table 2. Initial value and variation range of main structural parameters of motor
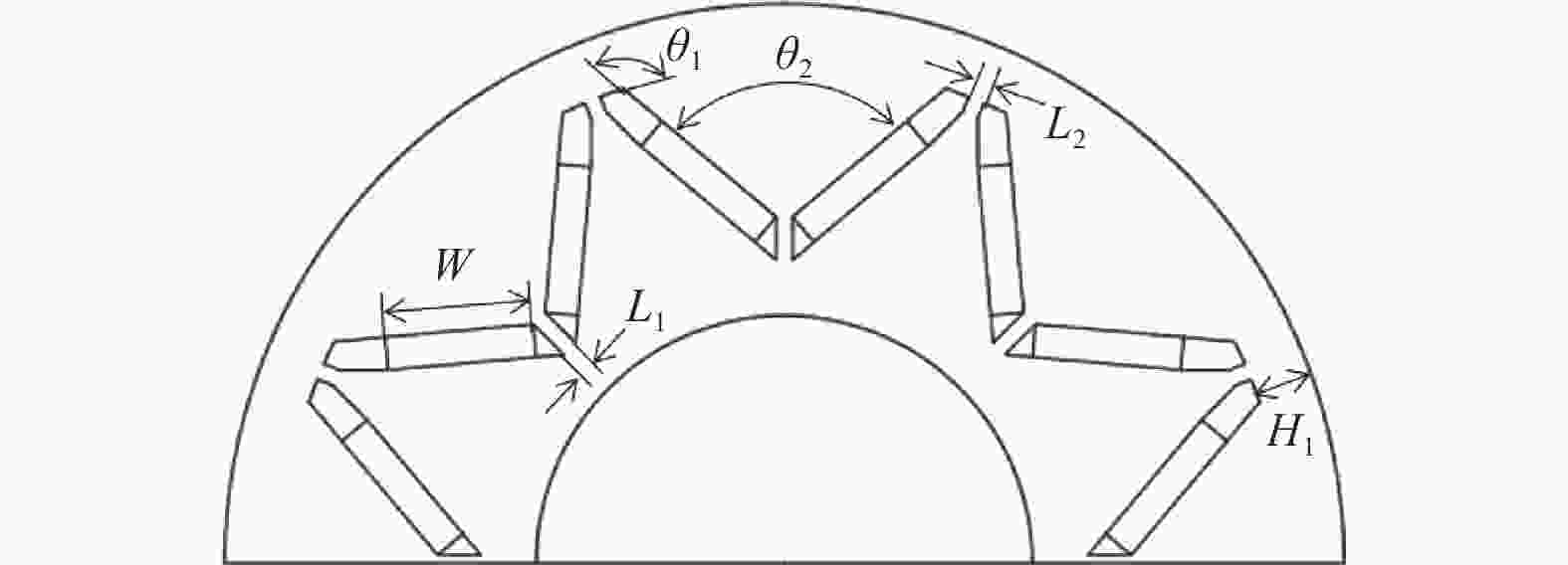
结构参数 初值 变化范围 磁桥厚度H1/mm 3 2～4 槽机械角$\theta_{1} $/(°) 150 130～170 磁槽角度$\theta_{2} $/(°) 180 100～180 隔磁桥间距L1/mm 0 0～2 槽间间隔L2/mm 1 0.8～1.2 永磁体宽度W/mm 18 17.5～18.5
下载: 导出CSV
表 3 结构参数灵敏度分析结果
Table 3. Sensitivity analysis results of structural parameters
结构参数 输出转矩 转矩脉动 齿槽转矩 综合灵敏度 磁桥厚度$H_{1} $/mm −0.26 −0.58 −0.98 0.61 槽机械角$\theta_{1} $/(°) −0.29 −4.00 −7.61 3.97 磁槽角度$\theta_{2} $/(°) 0.22 0.56 0.47 0.43 隔磁桥间距$L_{1} $/mm −0.07 −0.11 −0.54 0.23 槽间间隔$L_{2} $/mm −0.01 0.46 0.63 0.37 永磁体宽度W/mm 0.51 6.07 10.22 5.65
下载: 导出CSV
表 4 高灵敏度参数Kriging模型预测精度
Table 4. Prediction accuracy of Kriging model with high sensitivity parameters
目标 ME（≤0.2） RMSE（≤0.2） $R^{2} $（≥0.9） $y_{1} $ 0.06 0.08 0.93 $y_{2} $ 0.06 0.07 0.93 $ y_{3} $ 0.07 0.07 0.94
下载: 导出CSV
表 5 高灵敏度参数优化方案
Table 5. Optimization scheme of high sensitivity parameters
结构参数 $H_{1} / \mathrm{mm} $ $\theta_{1} /\left(^{\circ}\right) $ $W / \mathrm{mm}$ $L_{1} / \mathrm{mm}$ $L_{2} / \mathrm{mm}$ $\theta_{2} /\left(^{\circ}\right) $ 初始值 3.0 150.0 18.0 0 1.0 180 优化值 2.4 158.8 17.6 0 1.0 180
下载: 导出CSV
表 6 高灵敏度参数优化结果
Table 6. Optimization results of high sensitivity parameters
参数 初始结果 优化结果 优化效果% $ T_{\mathrm{out}} / \left( \mathrm{N} \cdot \mathrm{m}\right) $ 100.94 103.73 2.8 $T_{\mathrm{ri}} /{\text{%}}$ 7.73 6.50 −15.9 $T_{\mathrm{cog}} / \left( \mathrm{N} \cdot \mathrm{m}\right) $ 1.40 0.43 −69.3
下载: 导出CSV
表 7 低灵敏度参数优化方案
Table 7. Optimization scheme of low sensitivity parameters
结构参数 $H_{1} / \mathrm{mm} $ $\theta_{1} /\left(^{\circ}\right) $ $W / \mathrm{mm}$ $L_{1} / \mathrm{mm}$ $L_{2} / \mathrm{mm}$ $\theta_{2} /\left(^{\circ}\right) $ 优化前 2.4 158.8 17.6 0 1.0 180 优化后 2.4 158.8 17.6 0 0.8 180
下载: 导出CSV
表 8 低灵敏度参数优化结果
Table 8. Optimization results of low sensitivity parameters
参数 初始结果 优化结果 优化效果% $ T_{\mathrm{out}} / \left( \mathrm{N} \cdot \mathrm{m}\right) $ 103.73 103.65 −0.1 $T_{\mathrm{ri}} /{\text{%}}$ 6.50 5.27 −18.9 $T_{\mathrm{cog}} / \left( \mathrm{N} \cdot \mathrm{m}\right) $ 0.43 0.22 −48.8
下载: 导出CSV
表 9 单层优化Kriging模型预测精度
Table 9. Prediction accuracy of single-layer optimized Kriging model
目标 ME(≤0.2） RMSE(≤0.2） R2(≥0.9) $y_{1} $ 0.03 0.04 0.97 $ y_{2}$ 0.06 0.08 0.92 $ y_{3}$ 0.05 0.06 0.95
下载: 导出CSV
表 10 分层及单层优化方案比较
Table 10. Comparison of hierarchical and single-layer optimization schemes
结构参数 $H_{1} / \mathrm{mm} $ $\theta_{1} /\left(^{\circ}\right) $ $W / \mathrm{mm}$ $L_{1} / \mathrm{mm}$ $L_{2} / \mathrm{mm}$ $\theta_{2} /\left(^{\circ}\right) $ 初始值 3.0 150.0 18.0 0 1.0 180 分层优化 2.4 158.8 17.6 0 0.8 180 单层优化 2.6 151.0 17.7 0.8 0.9 167
下载: 导出CSV
表 11 不同优化方案对比
Table 11. Comparison of different optimization schemes
参数 初始结果 分层优化 单层优化 结果 优化效果/% 结果 优化效果% $ T_{\mathrm{out}} / \left( \mathrm{N} \cdot \mathrm{m}\right) $ 100.94 103.65 2.7 101.30 0.4 $T_{\mathrm{ri}} /{\text{%}}$ 7.73 5.27 −31.8 6.75 −12.7 $T_{\mathrm{cog}} / \left( \mathrm{N} \cdot \mathrm{m}\right) $ 1.40 0.22 −84.3 1.28 −8.6
下载: 导出CSV
-
[1] 郑江, 代颖, 石坚. 车用永磁同步电机的电磁噪声特性[J] . 电工技术学报,2016,31(S1):53 − 59. [2] 王硕, 康劲松, 钟再敏, 等. 电动汽车用永磁同步电机转矩脉动抑制方法综述[J] . 电源学报,2016,14(5):24 − 32. [3] 刘珅, 高琳. 永磁同步电机的改进模型预测直接转矩控制[J] . 电机与控制学报,2020,24(1):10 − 17. [4] 余洋, 从乐瑶, 田夏, 等. 定子电流矢量定向下PMSM转矩脉动抑制方法[J] . 中国电机工程学报,2019,39(11):3372 − 3382. [5] MORON C, GARCIA A, TREMPS E, et al. Torque control of switched reluctance motors[J] . IEEE Transactions on Magnetics,2012,48(4):1661 − 1664. doi: 10.1109/TMAG.2011.2173169 [6] MIR S, ELBULUK M E. Torque-ripple minimization in switched reluctance motors using adaptive fuzzy control[J] . Industry Applications IEEE Transactions on,1999,35(2):461 − 468. doi: 10.1109/28.753642 [7] NISHIO T , RYOSUKE Y , MASAHIKO K , et al. A method of torque ripple reduction by using harmonic current injection in PMSM[C]//Proceedings of 2019 IEEE 4th International Future Energy Electronics Conference (IFEEC). Singapore: IEEE, 2019. [8] 王凯, 孙海阳, 张露锋, 等. 永磁同步电机转子磁极优化技术综述[J] . 中国电机工程学报,2017,37(24):7304 − 7317. [9] 王晓远, 张力, 许卫刚. 基于田口算法的内置式永磁同步电机多目标优化设计[J] . 微电机,2016,49(5):1 − 5. doi: 10.3969/j.issn.1001-6848.2016.05.001 [10] 刘国海, 王艳阳, 陈前. 非对称V型内置式永磁同步电机的多目标优化设计[J] . 电工技术学报,2018,33(S2):385 − 393. [11] 曹永娟, 冯亮亮, 毛瑞, 等. 轴向磁场永磁记忆电机多目标分层优化设计[J] . 中国电机工程学报,2021,41(6):1983 − 1992. [12] 李祥林, 李金阳, 杨光勇, 等. 电励磁双定子场调制电机的多目标优化设计分析[J] . 电工技术学报,2020,35(5):972 − 982. [13] KAMAL C, THYAGARAJAN T, SELVAKUMARI M, et al. Cogging torque minimization in brushless DC motor using PSO and GA based optimization[C]//Proceedings of 2017 Trends in Industrial Measurement and Automation (TIMA). Chennai: IEEE, 2017: 1−5. [14] GONG J, GILLON F, CANH J T, et al. Proposal of a Kriging output space mapping technique for electromagnetic design optimization[J] . IEEE Transactions on Magnetics,2017:1. [15] CHO D H, JUNG H K. Multiobjective optimal design of interior permanent magnet synchronous motors considering improved core loss formula[J] . Energy Conversion IEEE Transactions on,1999,14(4):1347 − 1352. doi: 10.1109/60.815071 [16] YI W, DAN M I, RALLABANDI V, et al. Large-scale optimization of synchronous reluctance machines using CE-FEA and differential evolution[J] . IEEE Transactions on Industry Applications,2016,52(6):4699 − 4709. doi: 10.1109/TIA.2016.2591498 [17] LI F, HUA W, TONG M H, et al. Nine-phase flux-switching permanent magnet brushless machine for low-speed and high-torque applications[J] . IEEE Transactions on Magnetics,2015,51(3):1 − 4. [18] GAO J, DAI L T, ZHANG W J. Improved genetic optimization algorithm with subdomain model for multi-objective optimal design of SPMSM[J] . CES Transactions on Electrical Machines and Systems,2018,2(1):160 − 165. doi: 10.23919/TEMS.2018.8326463 [19] 张璐璐, 闫业翠, 马其华, 等. 电动汽车轮毂电机磁—热双向耦合研究[J] . 机电工程,2019,36(4):407 − 412. doi: 10.3969/j.issn.1001-4551.2019.04.013 [20] 徐磊, 朱孝勇, 张超, 等. 磁极径向组合轴向磁场永磁电机转矩品质分析与优化设计[J] . 中国电机工程学报,2021,41(6):1971 − 1983. [21] 马安琪. 增强型磁场调制直线永磁电机及其多目标优化设计[D]. 镇江: 江苏大学, 2019. [22] 杨国龙, 黄开胜, 赖文海, 等. 小型电动车用外转子永磁同步电动机的设计与分析[J] . 微电机,2015,48(10):11 − 14. doi: 10.3969/j.issn.1001-6848.2015.10.003 [23] 陈前, 廖继红, 赵文祥, 等. 低成本辅助凸极式辐向永磁电机的鲁棒性优化设计[J] . 中国科学:技术科学,2021,51(6):659 − 672. [24] 徐龙江, 牟磊, 汤磊, 等. 基于响应面模型与遗传算法的无轭分块电枢轴向磁场永磁电机齿槽转矩优化[J] . 微电机,2020,53(12):22 − 28. doi: 10.3969/j.issn.1001-6848.2020.12.005 [25] 李晓华, 黄苏融, 李良梓. 电动汽车用永磁同步电机振动噪声的计算与分析[J] . 电机与控制学报,2013,17(8):37 − 42. doi: 10.3969/j.issn.1007-449X.2013.08.006 [26] 钱喆, 唐光华, 王群京, 等. 转子结构优化削弱车用永磁同步电机振动噪音[J] . 电机与控制学报,2021,25(10):122 − 129. -
下载:
点击查看大图
计量
- 文章访问数: 301
- HTML全文浏览量: 647
- PDF下载量: 73
- 被引次数: 0
