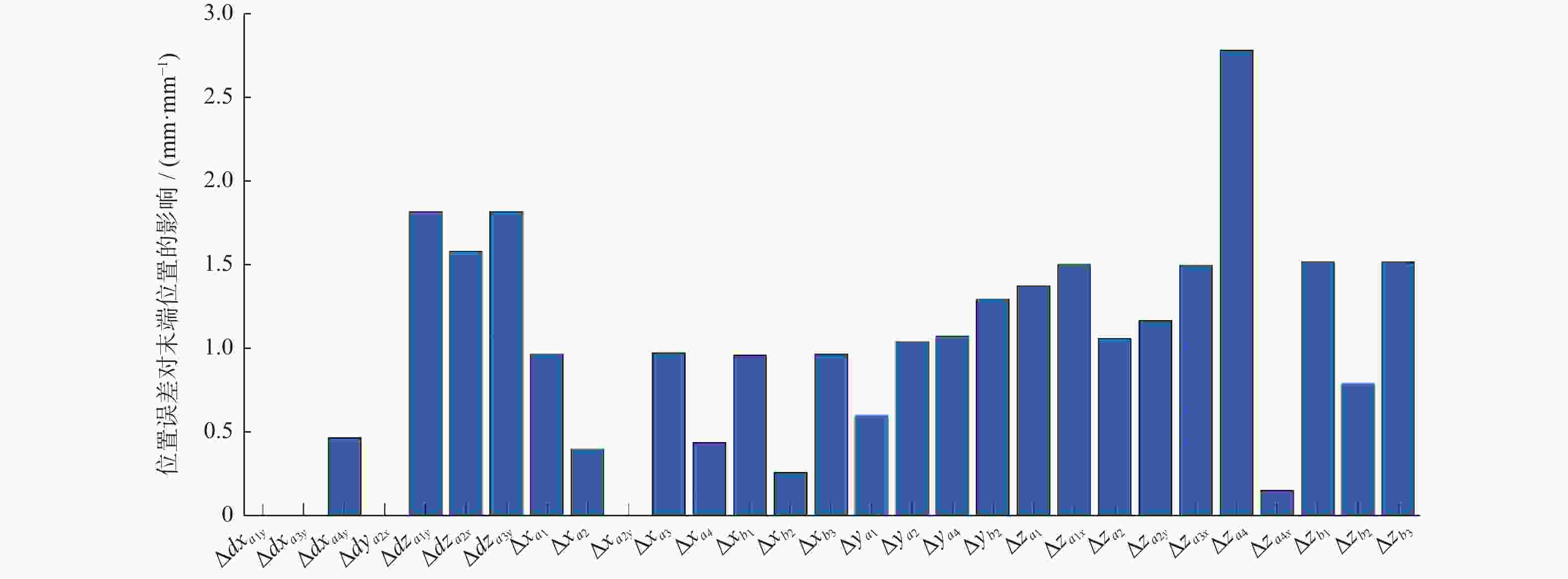
Error model and geometric error sensitivity simulation of new 2UPR/UPS/UP mechanism
-
摘要:
研究推导2UPR/UPS/UP+AC摆混联机构的解析反解,并以2UPR/UPS/UP为研究对象,系统阐述单关节误差模型和支链误差模型,并给出相关的几何误差源;建立被动误差、动平台位姿误差与各支链运动副误差源之间的误差映射模型,并基于机构误差模型进行各运动副误差源的误差灵敏度仿真分析. 研究成果可为基于2UPR/UPS/UP并联机构的五自由度混联机器人工程设计提供运动副误差分配及精度设计的理论支撑,同时为其他混并联机构的误差建模和灵敏度分析提供借鉴.
Abstract:The analytical inverse solution of 2UPR/UPS/UP+AC pendulum hybrid mechanism was derived. With 2UPR/UPS/UP mechanism as the research object, the single-joint error model and branched-chain error model were systematically expounded , and the related geometric error sources were given. The error mapping models among the passive error, the pose error of the moving platform and the error sources of the motion pairs of each branch chain were established, and the error sensitivity of the error sources of each motion pair was simulated based on the mechanism error model. The research results can provide theoretical support for motion error distribution and precision design of 5-DOF hybrid robot engineering design based on 2UPR/UPS/UP parallel mechanism, as well as reference for error modeling and sensitivity analysis of other hybrid mechanisms and parallel mechanisms.
-
Key words:
- 5-DOF hybrid robot /
- parallel mechanism /
- error model /
- error sensitivity analysis /
- passive error
-
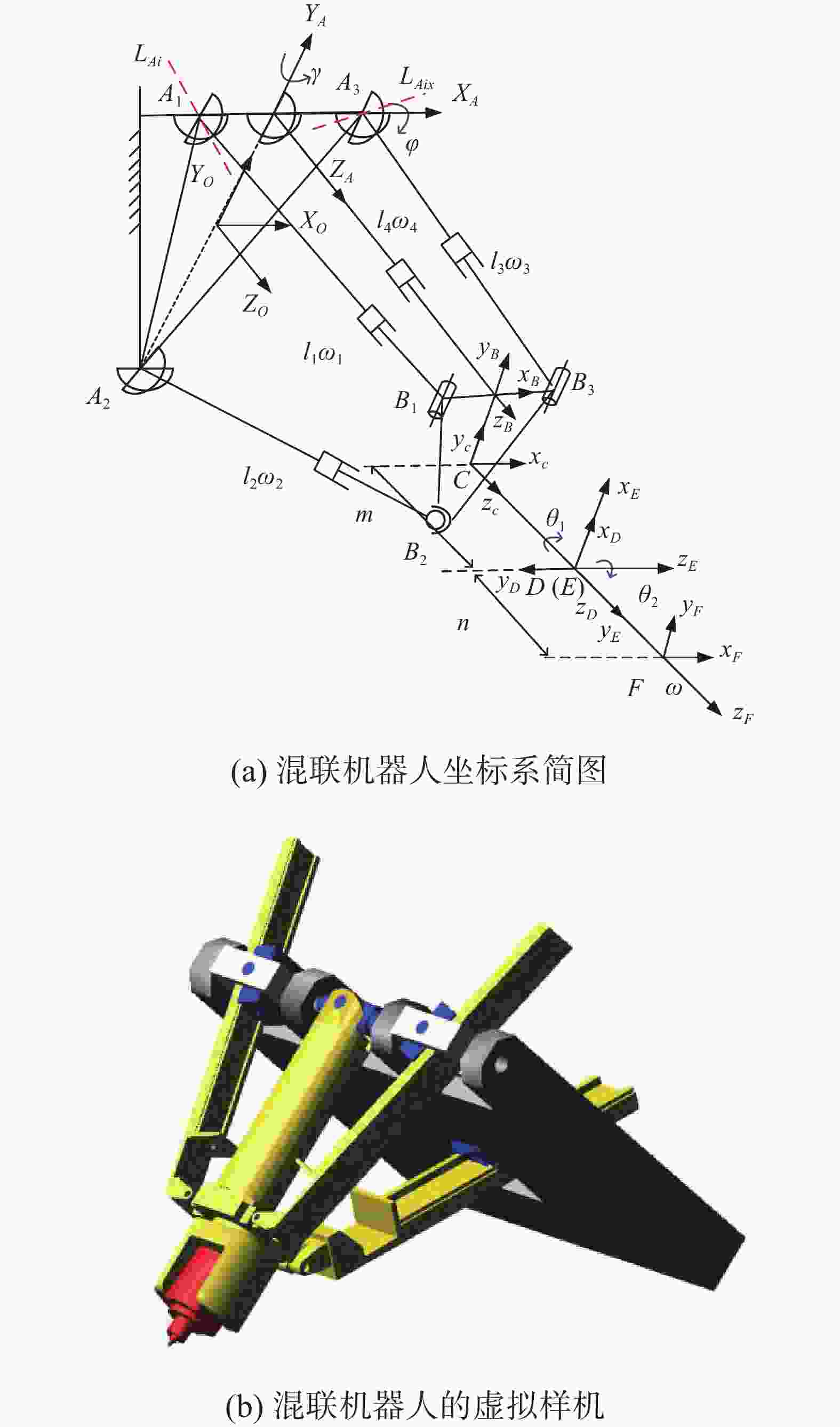
表 1 AC摆的DH参数
Table 1. DH parameters of AC pendulum
连杆 ${\alpha _{i - 1}}$ ${a_{i - 1}}$ ${d_i}$ ${\theta _i}$ 变化范围 1(D) 0º 0 m ${\theta _1}$ $\left[ {- {\text{π} },{\text{π} } } \right]$ 2(E) 0º 0 0 ${\theta _2}$ $\left[ {-{\text{π} } /2,{\text{π} } /2} \right]$ 3(F) 90º 0 n −90º 表 2 UPR支链几何误差定义
Table 2. Geometric error definition of UPR branch chain
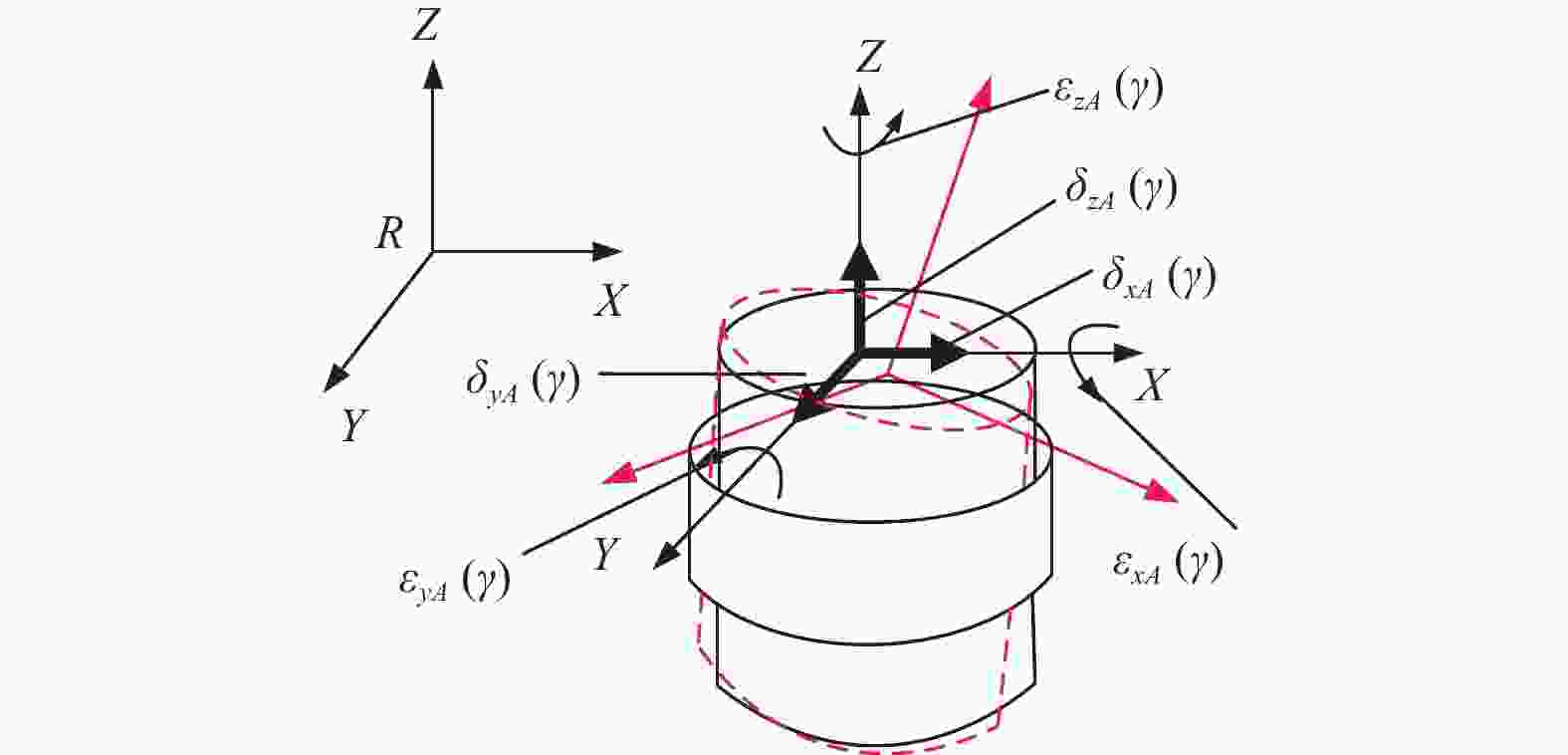
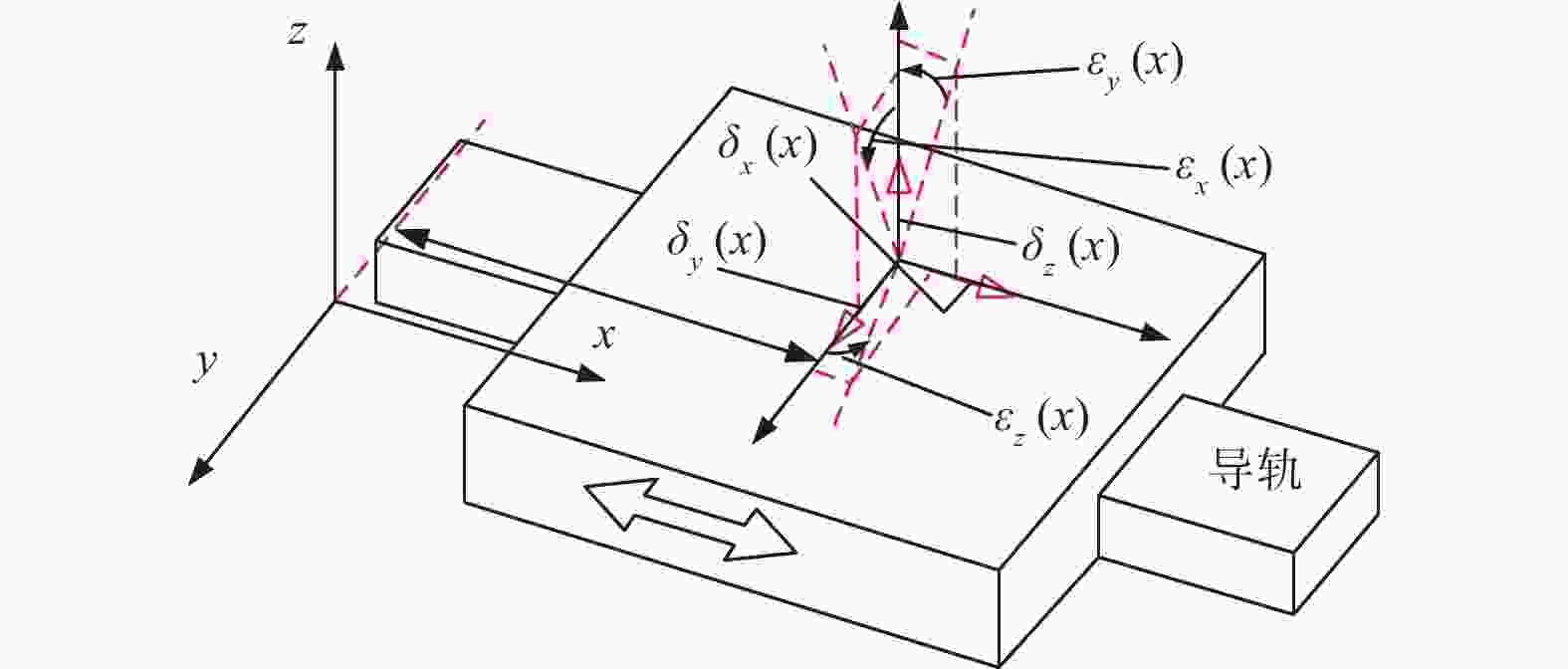
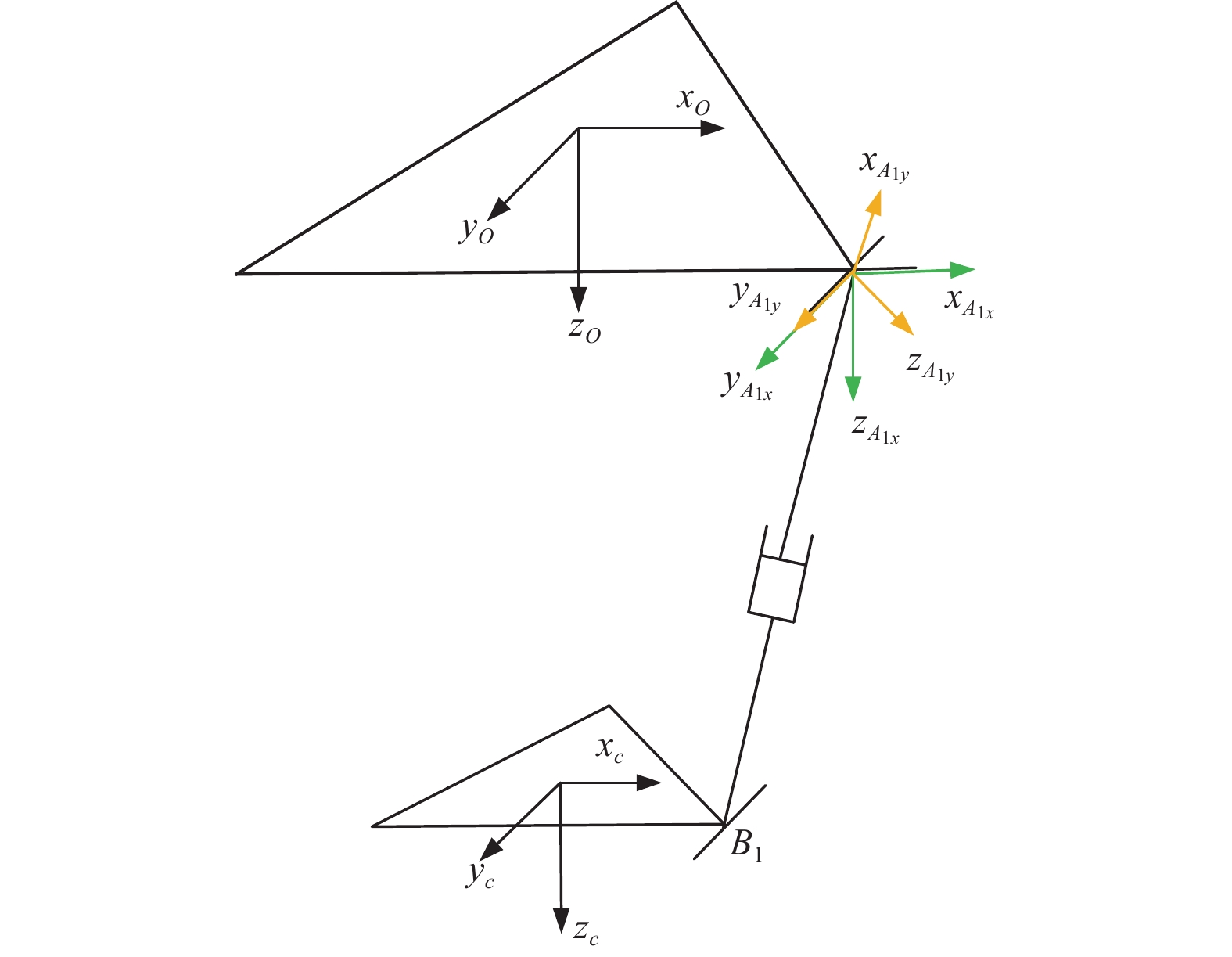
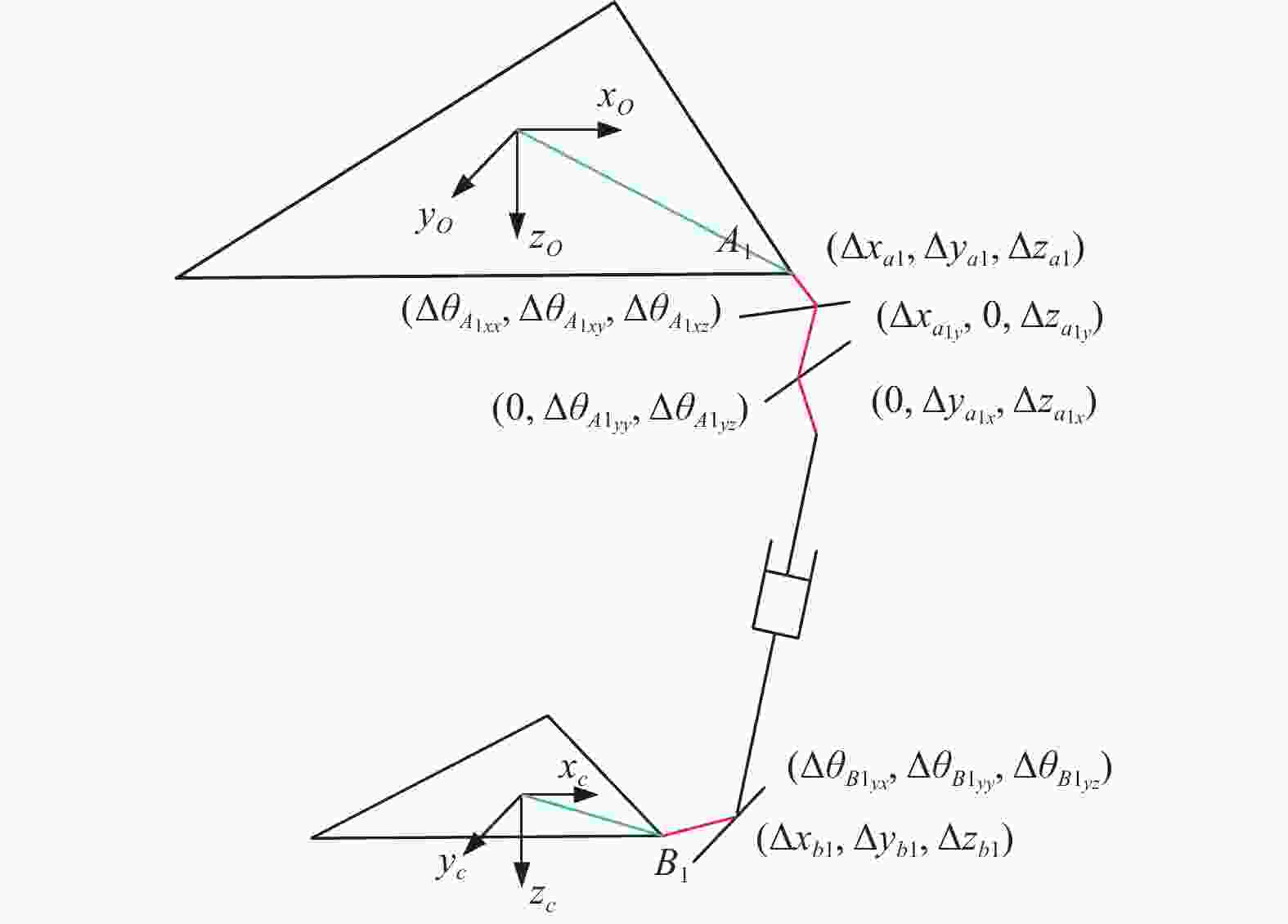
几何误差源 误差含义 备注 Δa1=
(Δxa1, Δya1, Δza1)U1副近架轴线位置误差 在系{O}下 ΔθA1x =
(ΔθA1xx, ΔθA1xy, ΔθA1xz)U1副近架轴线姿态误差 在系{O}下 Δa1x=
(Δxa1x, Δya1x, Δza 1x)U1副近架轴线与远
架轴线间位置误差在系{A1x}下 ΔθA1y =
(ΔθA1yx, ΔθA1yy, ΔθA1yz)U1副远架轴线姿态误差 在系{A1x}下 Δa1y=
(Δxa1y, Δya1y, Δza1y)U1副远架轴线与支
链轴线间位置误差在系{A1y}下 Δl1=
(Δxl1, Δyl1, Δzl1)支链移动方向误差 在系{A1y}下 ΔθB1y =
(ΔθB1yx, ΔθB1yy, ΔθB1yz)R1副旋转轴线姿态误差 在系{A1y}下 Δb1=
(Δxb1, Δyb1, Δzb1)动平台铰链点B1的位置误差 在系{C}下 表 3 并联机构杆长参数
Table 3. Rod length parameters of parallel mechanism
参数 ${l_1}/{\rm{mm}}$ ${l_2}/{\rm{mm}}$ ${l_3}/{\rm{mm}}$ Min 881 881 881 Max 1481 1481 1481 -
[1] 郭希娟, 黄天宇, 常福清. 动态式连接的少自由度混联机构的运动学分析[J] . 机械工程学报,2013,49(1):1 − 7. [2] BI Z M, JIN Y. Kinematic modeling of Exechon parallel kinematic machine[J] . Robotics and Computer-Integrated Manufacturing,2011,27(1):186 − 193. doi: 10.1016/j.rcim.2010.07.006 [3] HOSSEINI M A, DANIALI H M. Cartesian workspace optimization of Tricept parallel manipulator with machining application[J] . Robotica,2015,33(9):1948 − 1957. [4] 汪满鑫. 一种五自由度混联机器人静柔度建模与设计方法研究[D]. 天津: 天津大学, 2015. [5] 李林. 虚拟轴数控加工中心摆角精度的补偿[J] . 航空制造技术,2014,452(8):76 − 78. doi: 10.3969/j.issn.1671-833X.2014.08.018 [6] Li M, HUANG T, CHETWYND D G, et al. Forward position analysis of the 3DOF module of the TriVariant: A 5-DOF reconfigurable hybrid robot[J] . Journal of Mechanical Design,2006,128(1):319 − 322. doi: 10.1115/1.2125971 [7] DONG C, LIU H, LIU Q, et al. An approach for type synthesis of overconstrained 1T2R parallel mechanisms[C]//Proceedings of the 7th International Workshop on Computational Kinematics. Poitiers: Springer, 2017: 274-281. [8] YE H, WANG D, YUE Y, et al. Forward and inverse kinematics of a 5-DOF hybrid robot for composite material machining[J] . Robotics and Computer Integrated Manufacturing,2020,65:101961. doi: 10.1016/j.rcim.2020.101961 [9] 赵新华, 李彬. 6SPS并联机器人机构误差综合[J] . 中国机械工程,2009,20(18):2223 − 2226. doi: 10.3321/j.issn:1004-132X.2009.18.017 [10] 余跃庆, 田浩. 运动副间隙引起的并联机器人误差及其补偿[J] . 光学精密工程,2015,23(5):1331 − 1339. [11] 唐越, 郑金辉, 王庆杰. 六自由度工业机器人末端定位误差参数辨识与实验研究[J] . 现代制造工程,2021(2):21 − 26. [12] 徐卫良. 机器人机构误差建模的摄动法[J] . 机器人,1989(6):39 − 44,48. doi: 10.3321/j.issn:1002-0446.1989.06.001 [13] PATEL A J, EHMANN K F. Volumetric error analysis of a stewart platform-based machine tool[J] . CIRP Annals,1997,46(1):287 − 290. doi: 10.1016/S0007-8506(07)60827-0 [14] KUMAR A, WALDRON J. Numerical plotting of surfaces of positioning accuracy of manipulators[J] . Mechanism and Machine Theory,1981,16(4):361 − 368. doi: 10.1016/0094-114X(81)90010-0 [15] KIRIDENA V, FERREIRA P M. Mapping the effects of positioning errors on the volumetric accuracy of five-axis CNC machine tools[J] . International Journal of Machine Tools & Manufacture,1993,33(3):417 − 437. [16] 赵磊, 闫照方, 栾倩倩, 等. 大空间运动3-RRRU并联机器人运动学标定与误差分析[J] . 农业机械学报,2021,52(11):411 − 420. doi: 10.6041/j.issn.1000-1298.2021.11.045 [17] 洪振宇, 梅江平, 赵学满, 等. 可重构混联机械手—TriVariant的误差建模与灵敏度分析[J] . 机械工程学报,2006(12):65 − 69. doi: 10.3321/j.issn:0577-6686.2006.12.010 [18] 刘海涛, 潘巧, 尹福文, 等. TriMule混联机器人的精度综合[J] . 天津大学学报(自然科学与工程技术版),2019,52(12):1245 − 1254. [19] 谭兴强, 张键, 谢志江. 风洞6_PUS并联支撑机器人运动误差建模与补偿[J] . 农业机械学报,2014,45(4):334 − 340. doi: 10.6041/j.issn.1000-1298.2014.04.053 [20] 李寅翔. 2-UPR-RPU并联机构运动学标定研究[D]. 杭州: 浙江理工大学, 2016. [21] 李官明, 曲海波, 郭盛. 平面结构冗余并联机构的误差敏感度分析[J] . 机械工程学报,2020,56(23):45 − 57. [22] 于今, 余伟, 吴超宇, 等. 直线驱动型并联机器人误差模型与灵敏度分析[J] . 农业机械学报,2017,48(7):383 − 390. doi: 10.6041/j.issn.1000-1298.2017.07.049 [23] CHEN Y Z, XIE F G, LIU X J, et al. Error modeling and sensitivity analysis of a parallel robot with SCARA motions[J] . Chinese Journal of Mechanical Engineering,2014,27(4):693 − 702. doi: 10.3901/CJME.2014.0423.082 [24] 王海芳, 张恒, 皇甫一樊, 等. 码垛机器人运动精度可靠性及其灵敏度分析[J] . 中国工程机械学报,2016,14(6):475 − 480. -




 下载:
下载: